Index
Equilibrium Statistical Mechanics
Bipartite Spin Systems
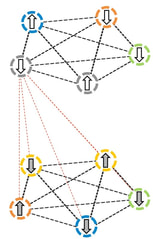
Consider a weighted combination of two lattices (in the case of the picture they are two fully connected graph). If you link the spins of each party of variables in a ferromagnetic or disorder way, you obtain a bipartite spin system. These kind of models present remarkable behaviour, with particular reference to the free energy which gives rise to a degenerate self-consistence equation. The model can be studied also outside the fully connected interaction between the parties, using, for instance, a Bernoulli distributed bond dilution. Moreover, it can be shown that an analogical neural network can be mapped into a bipartite model, widening, in this way, the possible applications of these kind of systems.
References A. Barra, G. Genovese, F. Guerra
J. Phys. A: Math. Theor. 44 245002 A. Barra, G. Del Ferraro, D. Tantari
EPJB (2013) 86:332 A. Barra, E. Agliari
J. Stat. Mech. (2011) P02027
Consider a weighted combination of two lattices (in the case of the picture they are two fully connected graph). If you link the spins of each party of variables in a ferromagnetic or disorder way, you obtain a bipartite spin system. These kind of models present remarkable behaviour, with particular reference to the free energy which gives rise to a degenerate self-consistence equation. The model can be studied also outside the fully connected interaction between the parties, using, for instance, a Bernoulli distributed bond dilution. Moreover, it can be shown that an analogical neural network can be mapped into a bipartite model, widening, in this way, the possible applications of these kind of systems.
References A. Barra, G. Genovese, F. Guerra
J. Phys. A: Math. Theor. 44 245002 A. Barra, G. Del Ferraro, D. Tantari
EPJB (2013) 86:332 A. Barra, E. Agliari
J. Stat. Mech. (2011) P02027
Non-equilibrium Statistical Mechanics
Current Large Deviations
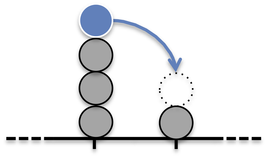
Take a chain in which each site can accommodate any number of particles. The probability of transition between the sites can depend on many parameters and the various models are classified with respect to them. This family of models is often called in the literature as Stochastic Particle Systems. For example, when the probability depends only on the number of particles of the departure site, the model is called Zero Range Process; if it decreases with the number of particles of the arrival site and increases with the number of particles of the departure site, we have the Misanthrope Process. Many properties of SPS have been described in the stationary regime, that is when observation times are large. However, it is also interesting to determine what is the behaviour of these models out of the stationary regime, that is what is the most typical of all the atypical configurations a system can assume. In particular, using the current as a physical observable, it is possible to study these behaviours from a microscopic as well as macroscopic perspective. Theoretical results can be confirmed using numerical simulations techniques stemming from large deviation theory, such as the Cloning Algorithm.
References P. Chleboun, S. Grosskinsky
JSP (2014) 154:432-465 S. Grosskinsky, G. M. Schutz, H. Spohn
JSP (2003) 113:389-410 M. R. Evans, B. Waclaw
J. Phys. A: Math. Theor. 47 095001
Take a chain in which each site can accommodate any number of particles. The probability of transition between the sites can depend on many parameters and the various models are classified with respect to them. This family of models is often called in the literature as Stochastic Particle Systems. For example, when the probability depends only on the number of particles of the departure site, the model is called Zero Range Process; if it decreases with the number of particles of the arrival site and increases with the number of particles of the departure site, we have the Misanthrope Process. Many properties of SPS have been described in the stationary regime, that is when observation times are large. However, it is also interesting to determine what is the behaviour of these models out of the stationary regime, that is what is the most typical of all the atypical configurations a system can assume. In particular, using the current as a physical observable, it is possible to study these behaviours from a microscopic as well as macroscopic perspective. Theoretical results can be confirmed using numerical simulations techniques stemming from large deviation theory, such as the Cloning Algorithm.
References P. Chleboun, S. Grosskinsky
JSP (2014) 154:432-465 S. Grosskinsky, G. M. Schutz, H. Spohn
JSP (2003) 113:389-410 M. R. Evans, B. Waclaw
J. Phys. A: Math. Theor. 47 095001
Network Optimisation
Clustering over Signed Graphs
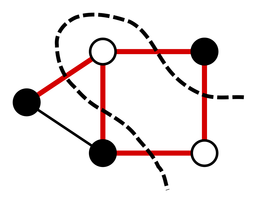
Consider a network where we associate to each edge a dichotomic variable, i.e. +1 or -1. In this way, we can build a signed adjacency matrix. Allowing the nodes to take +1 or -1 as well, it is interesting to determine the node configuration which maximizes the quadratic form associated to the graph. This can be achieved using many different techniques from Algebra, like spectral analysis, or Physics, like the Ginzburg-Landau functional. An important version of this problem is obtained when some values of the nodes are fixed a priori. This simple variation implies the breakdown of standard techniques and requires developing new optimization strategies.
References M. Cucuringu
Journal of Complex Networks, 3 (3):469-506 M. Cucuringu, A. Singer, D. Cowburn
Information and Inference: A Journal of the IMA, 1 (1), pp. 2167 M. Cucuringu, Y. Lipman , A. Singer
ACM Transactions on Sensor Networks, 8 (3), pp. 1-42
Consider a network where we associate to each edge a dichotomic variable, i.e. +1 or -1. In this way, we can build a signed adjacency matrix. Allowing the nodes to take +1 or -1 as well, it is interesting to determine the node configuration which maximizes the quadratic form associated to the graph. This can be achieved using many different techniques from Algebra, like spectral analysis, or Physics, like the Ginzburg-Landau functional. An important version of this problem is obtained when some values of the nodes are fixed a priori. This simple variation implies the breakdown of standard techniques and requires developing new optimization strategies.
References M. Cucuringu
Journal of Complex Networks, 3 (3):469-506 M. Cucuringu, A. Singer, D. Cowburn
Information and Inference: A Journal of the IMA, 1 (1), pp. 2167 M. Cucuringu, Y. Lipman , A. Singer
ACM Transactions on Sensor Networks, 8 (3), pp. 1-42
Quantitative Social Sciences
Immigration Phenomena and Interaction with Native Citizens
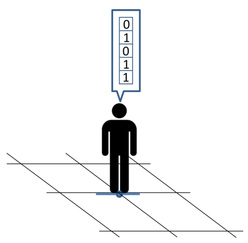
Statistical Mechanics (SM) is a tool which allows to describe physical situations in which many agents are present. The main idea is to divide people in two groups, immigrants and native citizens, and allow them to interact with each other. This corresponds to the typical scenario of a bipartite Curie-Weiss model, that is where two parties of spins are present. The above mentioned mathematical rationale is also able to manage different sociological indicators. Recently, typical SM techniques have been successfully applied to describe marriages among immigrants and native citizens in Spain, in order to understand possible trends of integration. Now, the idea is to apply those techniques to many other European States, even without considering the role of immigration: in this way it would be possible, in principle, to check the existence of cultural fractures among the native citizens of a country.
References A. Barra, P. Contucci
Europhysics Letters 89, 68001-68007 A. Barra, P. Contucci,. R. Sandell, C. Vernia
Nature Scientific Reports 3, 4174 E. Agliari, A. Barra, P. Contucci,. R. Sandell, C. Vernia
New Journal of Physics 16, 103034
Statistical Mechanics (SM) is a tool which allows to describe physical situations in which many agents are present. The main idea is to divide people in two groups, immigrants and native citizens, and allow them to interact with each other. This corresponds to the typical scenario of a bipartite Curie-Weiss model, that is where two parties of spins are present. The above mentioned mathematical rationale is also able to manage different sociological indicators. Recently, typical SM techniques have been successfully applied to describe marriages among immigrants and native citizens in Spain, in order to understand possible trends of integration. Now, the idea is to apply those techniques to many other European States, even without considering the role of immigration: in this way it would be possible, in principle, to check the existence of cultural fractures among the native citizens of a country.
References A. Barra, P. Contucci
Europhysics Letters 89, 68001-68007 A. Barra, P. Contucci,. R. Sandell, C. Vernia
Nature Scientific Reports 3, 4174 E. Agliari, A. Barra, P. Contucci,. R. Sandell, C. Vernia
New Journal of Physics 16, 103034